Proof that there is no Regular Map S3:{6,5}

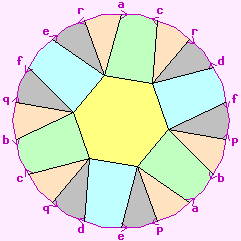
The figure shown here is not a regular map. Some of its holes have two edges, some have six.
This genus-3 map has five hexagonal faces, each meeting the same two neighbours three times each. It has six vertices and 15 edges.
Proof that there is no Regular Map S3:{6,5}

Suppose there is a regular map G3:{6,5}. It contains a hexagon, so we draw that. Then we make its vertices 5-valent. The result is seen to the left. Don't worry yet about where the edges go; they just go off the diagram, out of our field of view.

Now consider the adjacency graph of its faces. There are five of them, and each has six neighbours. The only regular way to arrange this is for each face to have only two distinct neighbours, bordering them on alternate edges. So we can colour in the five faces, as seen to the right.

Now it's time to think about how to join up the edges. Note that we have already drawn all six vertices, and six complete edges and 18 half edges; there is no more stuff to draw. All we have to do is join up the half edges in a way that makes the resulting map regular and orientable. The start of a tentative attempt at this is shown to the left.
There are relatively few distinct possible regular-looking ways to try joining up the half-edges; four, the way I count them. For each of these, the holes aren't all the same size, so the resulting map is not regular.
Other regular maps on the genus-3 oriented surface.
Index to other pages on regular maps.
Some pages on groups
Copyright N.S.Wedd 2009