|
Solid
|
Order
|
Group
|
Presentation
|
Cayley diagram
|
|---|
The Five Regular Platonic Polyhedra
|
|---|
|
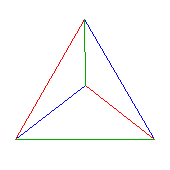
Tetrahedron
|
4
|
C2 × C2
C22
|
r g b
< r,g,b | r2, g2, b2, rg=b, (rg)2 >
|
|
|---|
|
Octahedron
|
6
|
D6 ≅ S3
|
k r g
< k,r,g | k2, r2, g2, rg=k, (rg)3 >
|

|
|---|
|
Cube
|
8
|
C2 × C2 × C2
C32
|
r g b
< r,g,b | r2, g2, b2, (rg)2, (gb)2, (br)2 >
|

|
|---|
|
D4
|
k r
< k,r | k4, r2, (kr)2 >
|

|
|---|
|
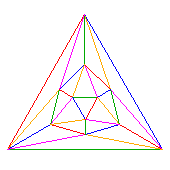
Icosahedron
|
12
|
A4
|
r g b
< r,g,b | r2, g3, b3, rbg >
If we add the rotations of the icosahedron (which form A5) to the above set of generators,
we get the Mathieu group M12.
|

|
|---|
|
This is not a group.
|
Unfortunately it this not the Cayley diagram of any group.
Starting at the top of the diagram,
rgb=1;
but from most vertices,
rgb
has period 8.
|
|
|
Dodecahedron
|
20
|
This is not a group.
|
Unfortunately it this not the Cayley diagram of any group.
If it were,
rg
gb
br
rb
bg and
gr
would all have period 10. Tracing out any of them
on the diagram forms a Hamiltonian cycle.
r g b
< r,g,b | r2, g2, b2,
(rg)10, (gb)10, (br)10 >
|

|
|---|
Truncated Polyhedra
|
|---|
|
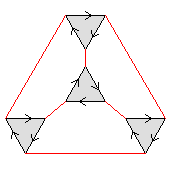
Truncated Tetrahedron
|
12
|
A4
|
k r
< r,g,b | k3, r2, (kr)3 >
|
|
|---|
|
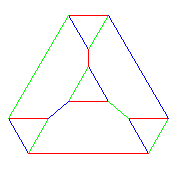
This is not a group.
|
This is a version of a diagram I found on the web. It purports to be a Cayley
diagram for A4. It implies that you can generate A4 with three generators each
of period 2: but A4 only has three elements of period 2, and they generate C2×C2.
|
|
|
Cuboctahedron
|
12
|
A4
|
r g
< r,g | r3, g3, (rg)2 >
|

|
|---|
|
Truncated Octahedron
|
24
|
S4
|
k r
< k,r | k4, r2, (kr)3 >
|

|
|---|
|
Truncated Octahedron
|
24
|
S4
|
b r g
< b,r,g | b2, r2, g2, (br)3, (rg)2, (gb)3 >
|

|
|---|
|
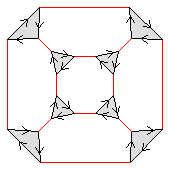
Truncated Cube
|
24
|
S4
|
k r
< k,r | k3, r2, (kr)4 >
|
|
|---|
|
Small Rhombicuboctahedron
|
24
|
S4
|
b r
< b,r | b4, r3, (br)2 >
|

|
|---|
|
Snub Cube
|
24
|
S4
|
r g b
< r,g,b | r2, g3, b2, rgb >
|

|
|---|
|
Icosidodecahedron
|
30
|
There is no group.
|
The groups with 30 elements are not interesting.
None of them could have a nice Cayley diagram such as this would have to be.
|

|
|---|
|
Truncated Cuboctahedron
|
48
|
S4 × C2
|
r g b
< r,g,b | r2, g2, b2,
(rg)2, (gb)3, (br)4 >
The central element is (rgb)3.
|

|
|---|
|
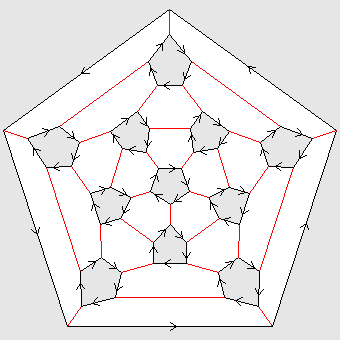
Truncated Icosahedron
|
60
|
A5
|
k r
< k,r | k5, r2, (kr)3 >
|
|
|---|
|
Truncated Dodecahedron
|
60
|
A5
|
k r
< k,r | k3, r2, (kr)5 >
|

|
|---|
|
Small Rhombicosidodecahedron
|
60
|
A5
|
r b
< b,r | b5, r3, (br)2 >
|

|
|---|
|
Sunb Dodecahedron
|
60
|
A5
|
k g r
< k,g,r | k5, g3, r2, kgr >
|

|
|---|
|
Great Rhombicosidodecahedron
|
120
|
A5 × C2
|
k r g
< k,r,b | k2, r2, g2,
(kr)2, (rg)3, (gk)5 >
|

|
|---|
Less regular Polyhedra
|
|---|
|
Prism
|
2N
|
DN |
k r
< k,r | k7, r2, krkr >
|

|
|---|
|
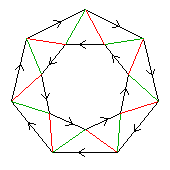
Antiprism
|
2N
|
DN |
k r g
< k,r,g | k7, r2, g2, kgr >
|
|
|---|
|
Pyritohedron
|
20
|
This is not a group.
|
This is equivalent to the dodecahedron, as can be seen by changing the angles until
the pentagons are more nearly regular. Again it is not the Cayley diagram of any group.
|

|
|---|
























