The Genus-2 Regular Map {8,3}

This genus-2 regular map, shown to the right, has six octagonal faces, of which three meet at each of its 16 vertices. It has 24 edges, and a Euler characteristic of -2.

Its Petrie polygons have 12 edges. Its Petrie dual is S³{12,3}.

Its dual is {3,8}. Its double cover is S³:{8,3}.

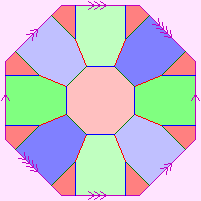
The diagram to the right may make its symmetry clearer. Note that the dark and the pale blue faces do not touch, nor the dark and pale red, nor the dark and pale green. Otherwise, each face borders each other face twice.

In this diagram the edges themselves have been coloured: each red edge separates a blue face from a green face, and has a dark red face at one end and a pale red face at the other end; and likewise for blue, and green, edges.

Antipodal Faces and Vertices

Each face is antipodal to one other face: dark red to pale red, etc. Each vertex is antipodal to one other vertex, as indicated by the black spots in the diagram to the right.

Another Portrayal

The diagram to the left shows the same map {8,3} in a less pleasing way.

Groups

This regular map can be used to draw Cayley graphs for the Pauli group and for GL(2,3): see Some Cayley Diagrams drawn on the surface of Genus 2.