The Genus-2 Map {9,3}

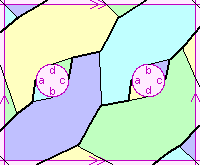
This map is not regular. It has 12 vertices, four nonagonal faces, and 18 edges.

If it were regular, its rotational symmetry group would be C₂²⋊C9, the central extension of A4 by C3.

Its dual is {3-9}, and can be constructed by pyritifying S²{4,6}.

One of its Petrie polygons is a Hamiltonian dodecagon – if we embed the sructure in three-space it forms a trefoil knot. It is shown in the diagram above right with thicker lines. The other Petrie polygons are all squares. Its Petrie dual, in the projective plane, is shown to the left.

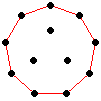
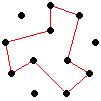
Regarded as a graph, it is the Möbius ladder of six rungs, as shown to the right. The four nonagonal faces of the map {9,3} are subgraphs, as shown below.