Other regular maps on the genus-3 oriented surface.
Index to other pages on regular maps.
Some pages on groups
Copyright N.S.Wedd 2009
S3:{7,3} is a regular map with 24 heptagonal faces, three meeting at each of its 56 vertices, and 84 edges. Its rotational symmetry group is PSL(2,7).
This page considers what happens to it when we rotate it about the centre of one of its faces, fixing that face.
We start be describing what happens when we rotate a dodecahedron in a similar way.
The face whose centre we are rotating about remains in its plane, as does the opposite face. If we rotated our face clockwise through 2π/5, we find that the opposite face has rotated counterclockwise through 2π/5. The rotation is smooth and continuous, and acts on all parts of the dodecahedron: vertices, edges, and faces. After rotation through 2π/5, it looks as it did at the start. This is a symmetry operation of order 5. The dodecahedron's being embedded in Euclidean 3-space makes it clear that this must happen.
But S3:{7,3} does not embed nicely (retaining all its symmetry) in 3-space. A rotation acts continuously on its vertices, but not on its edges. If we rotate it through 2π, each vertex follows a continuous path, and there are eight of these paths, with seven vertices lying on each. The edges however cannot be followed as the structure rotates.
The lack of continuity is not peculiar to S3:{7,3}, it is a property of all regular maps except those in S0 and C1. It is not what I want to discuss here.
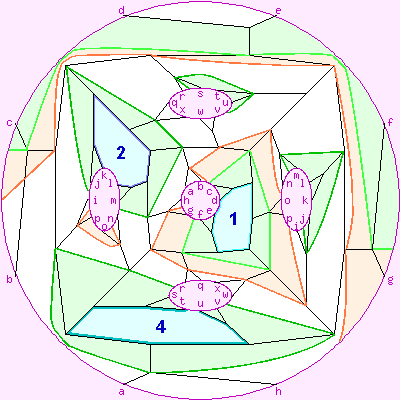
The diagram to the right is S3:{7,3}, coloured to show what happens as we rotate it about the centre of a face. When we rotate it clockwise about the face numbered 1:
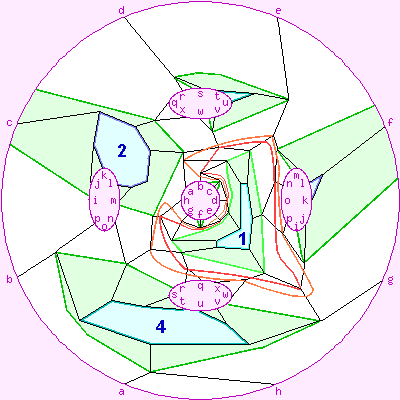 To the right is another version of the same diagram. In this version, the
S3:{7,3} has been redrawn slightly with respect to the "tunnels"
of the surface. Also, the orange circuit with 14 vertices on it has been
replaced ny two separate circuits, one shown in red and one in orange. When
the face numbered 1 is rotated one place clockwise, the vertices on
these two circuits each move one place along their own circuit.
To the right is another version of the same diagram. In this version, the
S3:{7,3} has been redrawn slightly with respect to the "tunnels"
of the surface. Also, the orange circuit with 14 vertices on it has been
replaced ny two separate circuits, one shown in red and one in orange. When
the face numbered 1 is rotated one place clockwise, the vertices on
these two circuits each move one place along their own circuit.
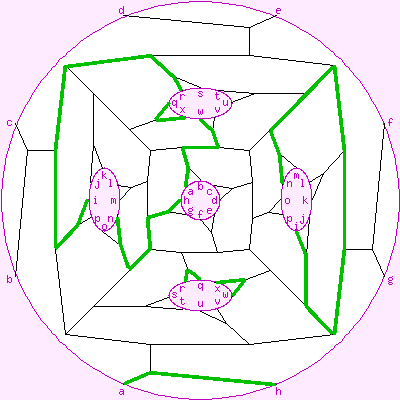
If we trace a path along the edges of S3:{7,3}, turning left, left, left, right, left, left, left, right, etc., at successive vertices, we obtain a 28-sided polygon such as the one shown to the left. There are 24 such polygons in all. A set of antipodes comprises three faces and three of these polygons.
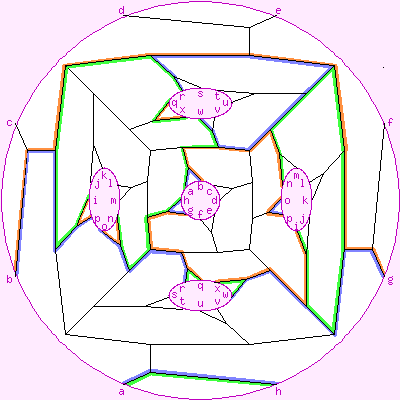
If we draw an antipodal set of three of these polygons, we find that any two of them have half of their edges in common, as shown to the right. Note that the orange polygon of this diagram runs close to the orange, and the orange and red, circuits of the two diagrams above it.
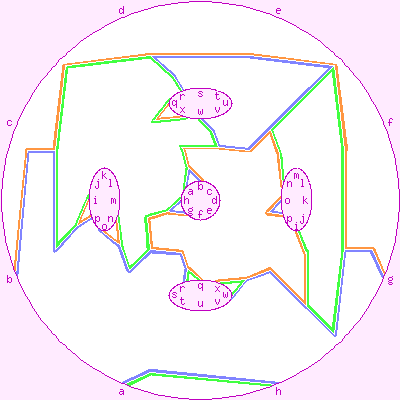
The diagram to the right has another interesting property. Suppose we consider only the polygons, and ignore the underlying S3:{7,3}, as shown to the left. Where all three polygons meet, place a vertex. If two of these vertices are directly connected by two coincident polygons, consider this as an edge. We now have the map S3:{14,3}, which is not quite regular.
Other regular maps on the genus-3 oriented surface.
Index to other pages on regular maps.
Some pages on groups
Copyright N.S.Wedd 2009