Holes and Petrie Polygons

A Petrie polygon is a polygon found in a regular map by travelling along its edges, turning sharp left and sharp right at alternate vertices.

A hole has a general definition, which applies to polytopes in any number of dimensions. But for our 2-dimensional purposes, we consider it as a polygon found in a regular map by travelling along its edges, taking the second-sharpest left at each vertex.

These concepts are related, and can be generalised as follows.
A 1st-order hole is just a face.
A 1st-order Petrie polygon is an ordinary Petrie polygon.
A 2nd-order hole is an ordinary hole.
A 2nd-order Petrie polygon is found by travelling along the edges of a regular map taking alternately the second-sharpest left and the second-sharpest right.
A 3rd-order hole is found by travelling along the edges of a regular map always taking the third-sharpest left.
A 3nd-order Petrie polygon is found by travelling along the edges of a regular map taking alternately the third-sharpest left and the third-sharpest right.
A 4th-order hole is found by travelling along the edges of a regular map always taking the fourth-sharpest left.
Etc.

This can be summarised:

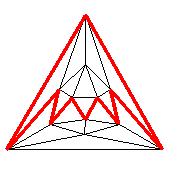
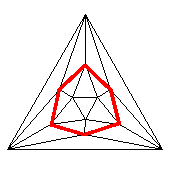
polygon	summary of route	minimum edges/vertex for this to be meaningful	symbol used in these pages
Vertex			V
Edge			E
Face	L,		F
Petrie polygon	L,R,	3	P
Hole	L2,	4	H
2nd-order Petrie polygon	L2,R2,	5	P₂
3rd-order hole	L3,	6	H₃
3rd-order Petrie polygon	L3,R3,	7	P₃
4th order Hole	L4,	8	H₄
4th-order Petrie polygon	L4,R4,	9	P₄
etc.

Icosahedron with one face shown in red.

Icosahedron with one Petrie polygon shown in red.

Icosahedron with one hole shown in red.

Icosahedron with one 2nd-order Petrie polygon shown in red.

We will use the abbeviations in the final column of the table when specifying the sets of antipodes of a polyhedron. Thus we would list the antipodal sets of the icosahedron as

(2V, 2H, P)	two vertices, two holes, one Petrie polygon
(2E)	two edges
(2F, P₂)	two faces, one 2nd-order Petrie polygon

Some regular maps
Some pages on groups