octahemioctahedron

Statistics

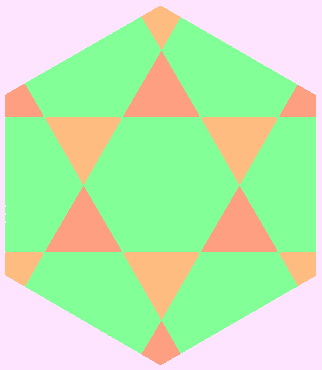
genus c	1, orientable
Schläfli formula c	{6,3}
V / F / E c	12 / 8+4 / 24
notes	This is not a regular map, it has faces of two kinds (it is quasiregular).
rotational symmetry group	A4×C2, with 24 elements
full symmetry group	S4×C2, with 48 elements

Relations to other Regular Maps

It is the result of rectifying {3,6}_(2,2).
It is the result of rectifying {6,3}_(2,2).

List of regular maps in orientable genus 1.

Comments

It can be immersed in ℝ³ as the octahemioctahedron.

Cayley Graphs based in this Regular Map

Type I

Other Regular Maps

General Index

The images on this page are copyright © 2010 N. Wedd