Regular Maps on the Klein bottle

I can find no regular map (as defined here) on the Klein bottle. Below are some non-regular maps.

First non-regular map

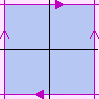
Consider the diagram to the right. It has one vertex, one face, and two edges.

It has one Petrie polygon, with four edges. It has three holes, one with two edges and two with one each.

It may appear to heve two holes, of one edge each, one running north and one running east from the vertex. But consider, first, the eastbound hole. We set off east from the vertex, planning to take the second left at each vertex. We come back to where we started, still planning to take the second left at each vertex. We have completed a one-edge hole. Alternatively, we can set off east from the vertex but planning to take the second right at each vertex. That produces another one-edge hole, comprising the same edge but being a different hole.

Now set off north from the vertex, planning to take the second left from each vertex. When we leave the top edge of the diagram, we reappear at the bottom edge, but now we are inverted and are planning to take the second right at each vertex. We reach the vertex, and continue, in a different state from our original state. After a second circuit, we return back where we started and in the same state that we started in. We have completed a two-edge hole.

So the east-west edge comprises two one-edge holes, while the north-south edge comprises one two-edge hole. These edges are different, and no symmetry element can map one to the other. The vertex is not regular, and so this is not a regular map.

Second non-regular map

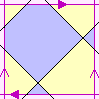
The diagram to the right has two vertices, two faces, and four edges.

It has one Petrie polygon with four edges and two each with two edges. It has two holes, each comprising the same path, with four edges.

Its face has two vertices (its north and south corners) at which the four-edge Petrie polygon continues to bound it, and two at which this Petrie polygon turns away from it. So its face is not regular, and it is not a regular map.

Third non-regular map

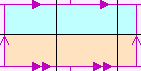
The diagram to the right also has two vertices, two faces, and four edges.

It has two Petrie polygons, each of four edges. It has four holes, each of two edges. This looks promising ...

However, two of its edges appear once in each Petrie polygon, while two each appear twice in one Petrie polygon. Each face is bounded by two of each kind of edge, and so is not regular. So this is not a regular map.

Moreover, each face borders itself on two edges and the other face on two edges, further proof that the faces are not regular.

Fourth non-regular map

The diagram to the right has four vertices, four faces, and eight edges.

It has two Petrie polygons each with eight edges. It has two holes each with four edges, and four holes each with two edges.

Each face has two edges which lie only in four-edge holes, and two which lie only in two-edge holes. So the faces, and the whole thing, are not regular.