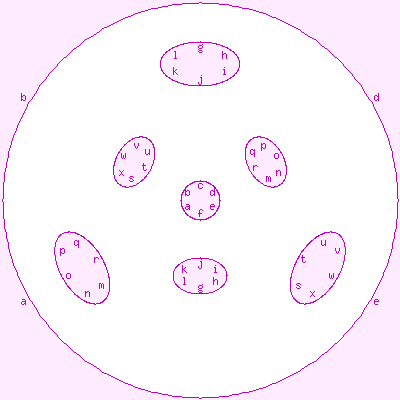
To draw these maps, we need a way of portraying the oriented genus-4 surface
in flat 2-space. We use the diagram shown to the right, which can be regarded
as an octahedron with a tunnel connected each pair of opposite faces.
This page shows just one (so far) of the regular maps that can be drawn on the genus-4 orientable manifold. For the purpose of these pages, a "regular map" is defined here.
To draw these maps, we need a way of portraying the oriented genus-4 surface
in flat 2-space. We use the diagram shown to the right, which can be regarded
as an octahedron with a tunnel connected each pair of opposite faces.
An image in the "thumbnail" column of the main table is a link to a page with more information about the regular map. Where the thumbnail image is shown on a grey background, the link is to a map that is not regular.
| Schläfli symbol | V+F-E=Eu | thumbnail (link) | dual | Rotational Symmetry Group | comments | qy |
|---|---|---|---|---|---|---|
| {12,3} | 24+6-36=-6 |  |
{3,12} |
A group of order 72 | Exists | 3 |
The things listed below are not regular maps.
| Schläfli symbol | V+F-E=Eu | thumbnail (link) |
|---|---|---|
| {3,9} | 24+8-36=-4 |  |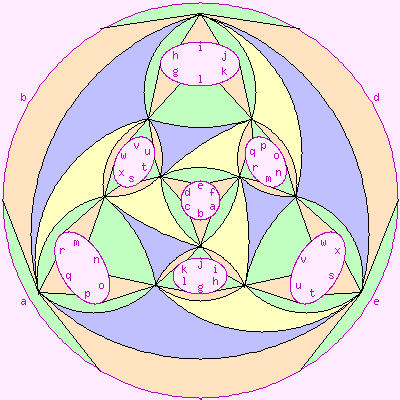
Index to other pages on regular maps;
indexes to those on
S0
C1
S1
S2
S3
S4.
Some pages on groups
Copyright N.S.Wedd 2009,2010