The Genus-C⁶ non-Regular map {20,4}

This is not a regular map. It is in genus-C⁶ (a sphere plus six crosscaps). It has one icosagonal face, meeting itself at all its 20 edges. It has one vertex and 10 edges, giving a Euler characteristic of -4.

Its dual is C⁶:{4,20}.

It has one Petrie polygon with 10 edges and two each with five edges. Each edge participates in the 10-sided Petrie polygon and in one five-sided one. Its holes have two edges.

The face shares all its vertices with itself. The face shares all its edges with itself. Some readers may consider that this invalidates it as a regular map.

If the five vertices are joined by a cycle of edges forming a pentagon, the map is converted to {5,6}, which is not regular. If a fifth vertex is added at the centre of the diagram, and connected to the existing ones by new edges, the map is converted to {6,5}, which is not regular.

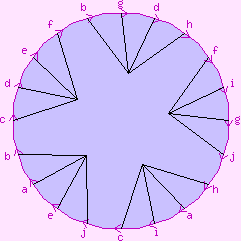
This map is similar in many respects to the above. But it differs in its holes, which have ten edges.

We can show that it is irregular by examining the hole (which is Eulerian and double-Hamiltonian). If we label the vertices P Q R S T, we find that the hole visits them in the order PSTRSQRPQT. Each vertex appears twice in this cycle, three places apart. So five of the edges appear midway between the two occurrences of some edge, and five don't; so it is not edge-transitive.

If the five vertices are joined by a cycle of edges forming a pentagon, the map is converted to a {5,6} which is not regular. If a fifth vertex is added at the centre of the diagram, and connected to the existing ones by new edges, the map is converted to a {6,5} which is not regular.

Its dual is C⁶:{4,20}.