Named Graphs
identified with regular maps |
not identified with regular maps |
known not to be regular
The things described in these pages as "regular maps" can be regarded as graphs
embedded in 2-manifolds. (I prefer to regard them as "regular polyhedra", but
that is not their usual name). Some of the more interesting graphs are
"named graphs".
If you are interested in a particular named graph, you can type its name into
the search box of your browser while viewing this page.
The page lists some named graphs, and for each which I know how to embed
regularly in some manifold, gives a link to a page where I show it as a
regular map.
Some named graphs can be embedded nicely in a manifold, but not in a fully regular
way. For example, the Franklin
graph can be embedded in the projective plane, where it is seen to be a truncated
hemi-octahedron, which is not face-transitive. A few of these are listed in the main
table of this page, but I have not tried to list irregular named graphs systematically.
Named Graphs identified with Regular Maps
Name of graph
(link to wikipedia) |
Picture of graph |
Valency |
Vertices |
Edges |
Full symmetry
group of graph |
Rotational
symmetry group of
regular map |
Schläfli symbol
(link to polyhedron) |
Picture of regular map |
Faces of regular map |
|---|
| Cube |
 |
3 |
8 |
12 |
48 S4×C2 |
24 S4 |
{4,3} |
 |
6 squares |
| Cycle graph |
 |
2 |
n |
n |
2n D2n |
n Cn |
{5,2} |
 |
2 pentagons |
| Desargues graph |
 |
3 |
20 |
30 |
240 S5×C2 |
60 A5 |
C6:{10,3} |
 |
6 decagons |
| Dodecahedron |
 |
3 |
12 |
30 |
120 S5 |
60 A5 |
{3,5} |
 |
12 pentagons |
| Dyck graph |
 |
3 |
32 |
48 |
192 |
96 |
{6,3}(4,4) |
 |
16 hexagons |
| 96 |
S3{8,3} |
 |
12 octagons |
/1/hex.php#4h4
| F26A graph |
 |
3 |
26 |
39 |
78 |
78 |
{6,3}(2,4) |
 |
13 hexagons |
| Franklin graph |
 |
3 |
12 |
18 |
48 S4×C2 |
26 S4 |
Not regular. Can be embedded in the projective plane, as a truncated hemi-octahedron, having .. |
.. 3 squares and 4 hexagons |
| Heawood graph |
 |
3 |
14 |
21 |
336 PGL(2,7) |
21 C7⋊C3 |
S3:{14,3} |
 |
Not quite regular. 3 14-gons |
| Icosahedron |
 |
3 |
20 |
30 |
120 A5×C2 |
60 A5 |
{3,5} |
 |
20 triangles |
| K1 |
 |
0 |
0 |
0 |
1 S1 |
1 S1 |
{0,0} |
 |
1 point-bounded face |
| K2 |
 |
1 |
2 |
1 |
2 S2 |
2 S2 |
{2,1} |
 |
1 digon |
| K3 |
 |
2 |
3 |
3 |
6 S3 |
6 S3 |
{3,2} |
 |
2 triangles |
| K4 |
 |
3 |
4 |
6 |
24 S4 |
12 A4 |
{3,3} |
 |
4 triangles |
| K5 |
 |
4 |
5 |
10 |
120 S5 |
20 C5⋊C4 |
{4,4}(2,1) |
 |
5 squares |
| K6 |
 |
5 |
6 |
15 |
720 S6 |
60 A5 |
{3,5} |
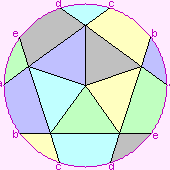 |
10 triangles |
| K7 |
 |
6 |
7 |
21 |
5,040 S7 |
42 C7⋊C6 |
{3,6}(1,3) |
 |
14 triangles |
| K3,3 Utility
or
Thomsen graph |
 |
3 |
6 |
9 |
72 (S3×S3)⋊C2 |
18 D6×C3 |
{6,3}(0,2) |
 |
3 hexagons |
| K4,4 |
 |
4 |
4 |
8 |
1,152 (S4×S4)⋊C2 |
16 C22⋊C4 |
{4,4}(2,2) |
 |
8 squares |
| 16 |
S3{8,4} |
 |
4 octagons |
| K2,2,2 |
 |
4 |
6 |
12 |
96 (S2×S2×S2)⋊S3 |
12 A4 |
{3,4} |
 |
8 triangles |
| K3,3,3 |
 |
6 |
9 |
27 |
1,296 (S3×S3×S3)⋊S3 |
54 |
{3,6}(3,3) |
 |
18 triangles |
| K4,4,4 |
 |
8 |
12 |
48 |
82,944 (S4×S4×S4)⋊S3 |
96 |
S3{8,3} |
 |
12 octagons |
| Möbius-Kantor graph |
 |
3 |
16 |
24 |
96 |
48 |
S2:{8,3} |
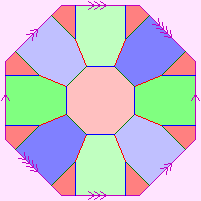 |
6 octagons |
| Nauru graph |
 |
3 |
24 |
36 |
144 S4×D6 |
72 |
{6,3}(0,4) |
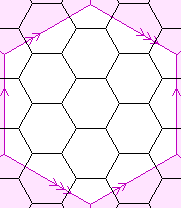 |
12 hexagons |
| Octahedron |
See K2,2,2 above |
| Paley order-13 graph |
 |
6 |
13 |
39 |
78 |
78 |
{3,6}(2,4) |
 |
26 triangles |
| Pappus graph |
 |
3 |
18 |
27 |
216 |
54 |
{6,3}(3,3) |
 |
9 hexagons |
| Petersen graph |
 |
3 |
10 |
15 |
120 S5 |
60 A5 |
C1:{5,3} |
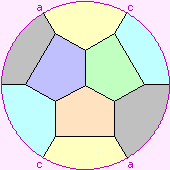 |
6 pentagons |
| Shrikhande graph |
 |
6 |
16 |
48 |
192 |
96 |
{3,6}(4,4) |
 |
32 triangles |
| Tetrahedron |
See K4 above |
Named Graphs not identified with Regular Maps
These either don't exist as regular maps, or do but I don't know how.
| Biggs-Smith graph |
 |
3 |
102 |
153 |
2448 PSL(2,17) |
? |
|
| Clebsch graph also called the Greenwood-Gleason graph |
 |
5 |
16 |
40 |
1920 |
Maybe related to S3:{4,5}
(irregular) |
(20 squares) |
| Coxeter graph |
 |
3 |
28 |
42 |
336 PGL(2,7) |
S2:{7,3}
(irregular)If S2:{7,3} existed as a regular polyhedron, then S3:{7,3} could be a double cover of it. |
(12 heptagons) |
| 10-crown |
 |
4 |
10 |
20 |
80 |
Maybe related to S2:{5,4}
(irregular) |
(8 pentagons, but this graph is bipartite) |
| Double-star snark |
 |
3 |
30 |
45 |
? |
maybe S7:{30,3} ? |
3 30-gons |
| Foster graph |
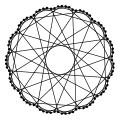 |
3 |
90 |
135 |
4320 |
? |
|
| Hall-Janko graph |
 |
? |
100 |
? |
? |
? |
|
| Higman-Sims graph |
 |
22 |
100 |
1100 |
88,704,000 HS⋊C2) |
? |
|
| Hoffman-Singleton graph |
 |
7 |
50 |
175 |
252,000 PSU(3,52)⋊C2 |
? |
|
| Horton graph |
 |
3 |
96 |
144 |
96 S4×C2×C2 |
Not the same as {6,3}(0,8) |
|
| K8 |
 |
7 |
8 |
28 |
40,320 S8 |
? |
|
| Tutte-Coxeter graph |
 |
3 |
30 |
45 |
1,440 Aut(S6) |
? |
|
Named Graphs known not to be Regular


































































