symbol
C&D no.
(link)
Group
N4.2′


N4.2
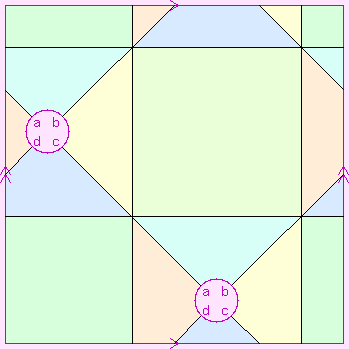
N4.1′

self

cantankerous
R4.1

This page shows some of the regular maps that can be drawn on the genus-4c (a sphere plus four crosscaps) non-orientable manifold. For the purpose of these pages, a "regular map" is defined here.
To draw these maps, we need a way of portraying this surface in 2-space. Pink letters show how the "cut edges" are to be joined up, and light pink regions are not part of the surface. This is further explained by the page Representation of 2-manifolds.
| Schläfli symbol C&D no. | V+F-E=Eu | thumbnail (link) | dual | Symmetry Group | comments | qy |
|---|---|---|---|---|---|---|
| {6,4}3 N4.2′ |
6+4-12=-2 |  |
next |
[48] |  |
2 |
| {4,6}3 N4.2 |
6+4-12=-2 |  |
prec. |
|||
| {6,4}6 N4.1′ |
6+4-12=-4 |  |
next self |
[48] |  cantankerous |
2 |
| {4,6}6 R4.1 |
4+6-12=-4 |  |
prec. |
The things listed below are not regular maps.
| {18,3} | 6+1-9=-2 |  |
{3,18} | D6 | ∄ See {3,18} for an unconvincing proof. |
| {3,18} | 1+6-9=-2 |  |
{18,3} The triangular prism | ||
| {8,3} | 16+6-24=-2 | {3,8} |
|||
| {3,8} | 61+6-24=-2 |  |
{8,3} |
Index to other pages on regular maps;
indexes to those on
S0
C1
S1
S2
C4
C5
S3
C6
S4.
Some pages on groups
Copyright N.S.Wedd 2009,2010