symbol
C&N no.
(link)
Group
N5.1′

?
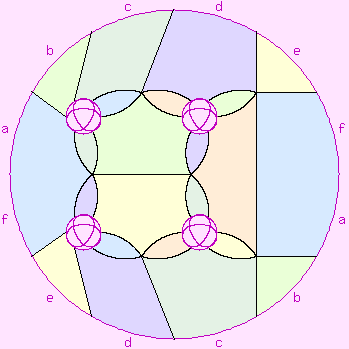
N5.1

?
N5.2′

?
N5.2
?
N5.3
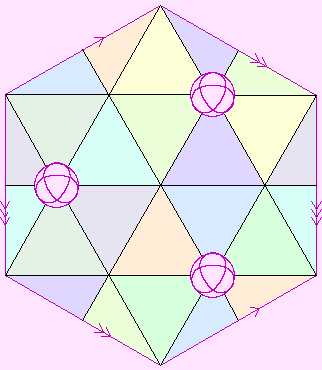
The hemi-icosahedron
N5.4

This page shows some of the regular maps that can be drawn on the genus-C5 (a sphere plus five crosscaps) non-orientable manifold. For the purpose of these pages, a "regular map" is defined here.
| Schläfli symbol C&N no. | V+F-E=Eu | thumbnail (link) | dual | Symmetry Group | comments | qy |
|---|---|---|---|---|---|---|
| {5,4} N5.1′ |
15+12-30=-4 |  |
{4,5} ? |
S5 | ? | 6 |
| {4,5} N5.1 |
12+15-30=-4 |  |
{5,4} ? |
|||
| {6,4} N5.2′ |
9+6-18=-4 |  |
{4,6} ? |
A group of order 72 | ? | 3 |
| {4,6} N5.2 |
6+9-18=-4 |  |
{6,4} ? |
|||
| {5,5} N5.3 |
6+6-15=-4 |  |
self-dual The hemi-icosahedron |
A5 | ? | 3 |
| {6,6} N5.4 |
3+3-9=-4 |  |
self-dual |
A group of order 36 | ? | 1½ |
Index to other pages on regular maps;
indexes to those on
S0
C1
S1
S2
C4
C5
S3
C6
S4.
Some pages on groups
Copyright N.S.Wedd 2009,2010